Subject to the constraint 2x2 (y 1)2 18 Solution We check for the critical points in the interior f x = 2x;f y = 2(y1) =)(0;May 26, · First, remember that graphs of functions of two variables, z = f (x,y) z = f ( x, y) are surfaces in three dimensional space For example, here is the graph of z =2x2 2y2 −4 z = 2 x 2 2 y 2 − 4 This is an elliptic paraboloid and is an example of a quadric surface We saw several of these in the previous sectionFeb 05, 13 · Find the maximum rate of change of f(x,y)= ln (x^2 y^2) at the point (1, 4) and the direction in which it occurs 1) Maximum rate of change = 2)

Find The Local Linear Approximation L To The Function F X Y Ln Xy At The Point P 1 2 Compare The Brainly In
F(x y)=ln(x^2+y^2)
F(x y)=ln(x^2+y^2)-Jul 05, 09 · For this one we need the log rule, chain rule and the power rule y = ln (x^2 y^2) dy/dx = 1/ (x^2 y^2) * (2x 2y (dy/dx)) dy/dx = (2x 2y dy/dx) / (x^2 y^2) We need to split the fraction so that all the dy/dx terms can be on the leftThe first derivative of mathz/math with respect to mathx/math is math\frac {\partial z}{\partial x}=\frac {2x}{x^2y^2}/math The second derivative is



Complex Analysis Gamelin Ii 5 Problems And Solutions Derivative Trigonometric Functions
(b) f(x,y) = 2x2 xy2 −2, D= {(x,y) ∈ R2;x2 y2 64} Solution (a) Calculating the partial derivatives gives f x = y 2 √ x − 1, f y = √ x−2y 6 Finding critical points f x = 0, f y = 0 =⇒ (x,y) = (4,4) Note that (4,4) ∈ Dand that f(4,4) = 12 The boundary of Dconsists of four line segments1) is a critical point The second derivative test f xx = 2;f yy = 2;f xy = 0 shows this a local minimum withMar 11, 07 · Show in 2 ways that the function ln(x^2y^2) is harmonic in every domain that does not contain the origin My problem with this quesiton is firstly, I don't know two ways, because normally I would do CauchyRiemann equations but there is no real/imaginary numbers so I don't know what is u(x,y) and what is v(x,y) As a function I know that if I differentiate in terms of x I get
Free multi variable limit calculator solve multivariable limits stepbystepGet an answer for 'Show that z=ln((x^2) (y^2)) is a solution of Laplace's equation δ^2z/δx^2 δ^2/δy^2 = 0' and find homework help for other Math questions at eNotesIf we have f (x, y) = \ln (x^2 y^2) Note that the y term is missing here, and using the chain rule with \ln, we get d/dx ~ (\ln (x^2 y^2)) = \dfrac {1} {x^2 y^2}~d/dx~ (x^2 y^2) = \dfrac {1} {x^2 y^2} (2x0) =\dfrac {2x} {x^2 y^2}
E y = x Then base e logarithm of x is ln(x) = log e (x) = y The e constant or Euler's number is e ≈ Ln as inverse function of exponential function The natural logarithm function ln(x) is the inverse function of the exponential function e x For x>0, f (f 1 (x)) = e ln(x) = x Or f 1 (f (x)) = ln(e x) = x NaturalApr , 21 · Find local max point of f (x,y) = ln (x^2 y^2 1) Use gradient descent method$f(x,y)$ is the composition of two functions If $g(x,y) = x^2 y^2$ and $h(s) = \ln(s)$ then $f(x,y) = h(g(x,y))$ $g(x,y)$ is continuous for all $x$ and $y$ What is the range of $g(x,y)?$ For what values of $s$ in the range of $g(x,y)$ is $h(s)$ not continuous?



Solved Evaluate Partial Differential U Partial Differenti Chegg Com



Find The Local Linear Approximation L To The Function F X Y Ln Xy At The Point P 1 2 Compare The Brainly In
F(x, y, z) = z tan −1 (y 2)i z 3 ln(x 2 4)j zk Find the flux of F across S, the part of the paraboloid x 2 y 2 z = 30 that lies above the plane z = 5 and is oriented upward S F · dS = Expert Answer Previous question Next question Get more help from Chegg Solve it with our calculus problem solver and calculatorFind dy/dx y = natural log of x^2y^2 y = ln (x2 y2) y = ln ( x 2 y 2) Differentiate both sides of the equation d dx (y) = d dx (ln(x2 y2)) d d x ( y) = d d x ( ln ( x 2 y 2)) The derivative of y y with respect to x x is y' y ′ y' y ′ Differentiate the right side of the equation Tap for more stepsAnswer to Let f(x, y) = ln (x^2 y^2) Which one of the following curves is a simple closed path in the domain of the function f ?
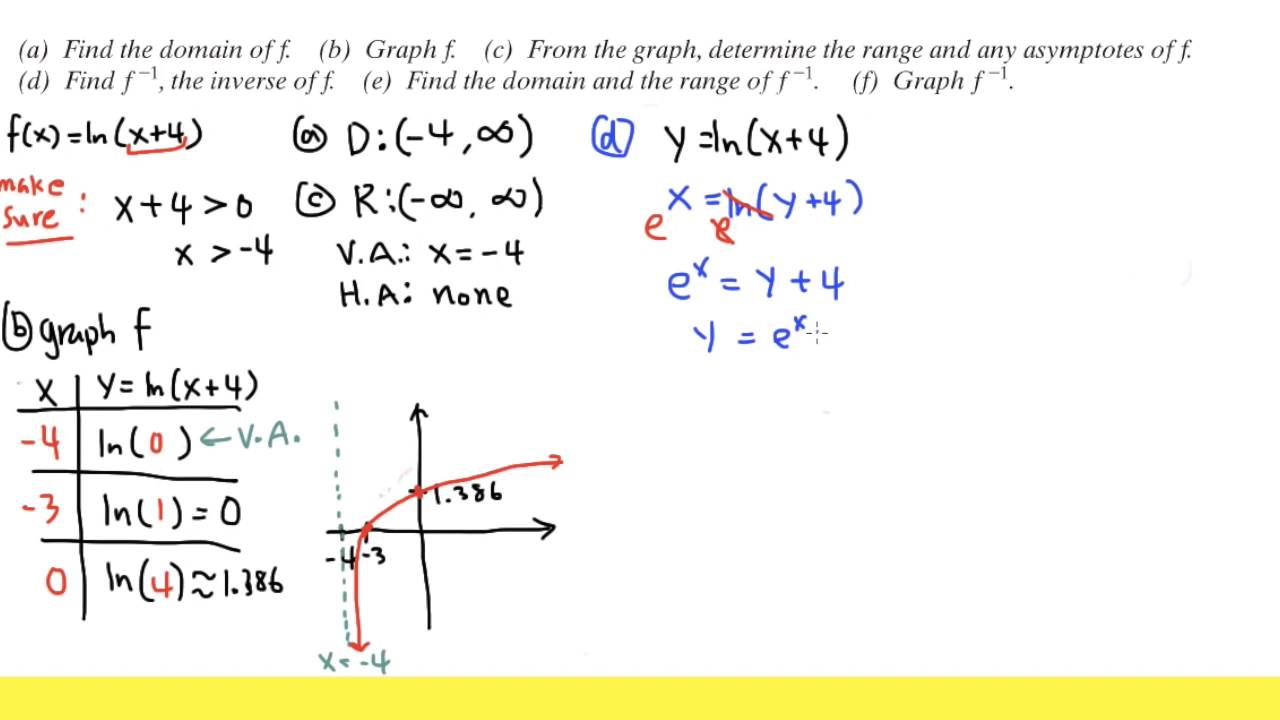


F X Ln X 4 Domain Range Graph And Its Inverse Youtube



Complex Analysis Gamelin Ii 5 Problems And Solutions Derivative Trigonometric Functions
Oct 13, 16 · Homework Statement for the domain of ln (x^2 y^2 ) , it it given in my notes that the ans is x ≠ 0 and y ≠ 0 IMO , it's wrong to give x ≠ 0 and y ≠ 0 , because the meaning of x ≠ 0 and y ≠ 0 is that x and y cant be 0 all the times so just leave the ans (x^2 y^2 ) > 0 , will do(a) f(x;y) = p x2 y2 1 ln(4 x2 y2) The function is de ned for x 2 y 1 0 and 4 x2 y2 0 The domain is D = f(x;y) 2R2j1 x2 y2 < 4g This is an annular region in the xyplane (b) f(x;y) = p x p y p x2 y2 16 The function is de ned for x 0, y 0, and x2 y2 16 0 The domain is D = f(x;y) 2R2jx 0;yF(x, y) = xyln(x2 y2) = rcosθrsinθln(r2) = r2lnrsin(2θ) = g(r, θ) The partial derivatives of g are given by ∂g ∂r = r(2lnr 1)sin(2θ), ∂g ∂θ = 2r2lnrcos(2θ) Since r > 0 and sin2θ > 0 in our range, the equation ∂g ∂r = 0 implies that 2lnr 1 = 0 or r = e − 1 2
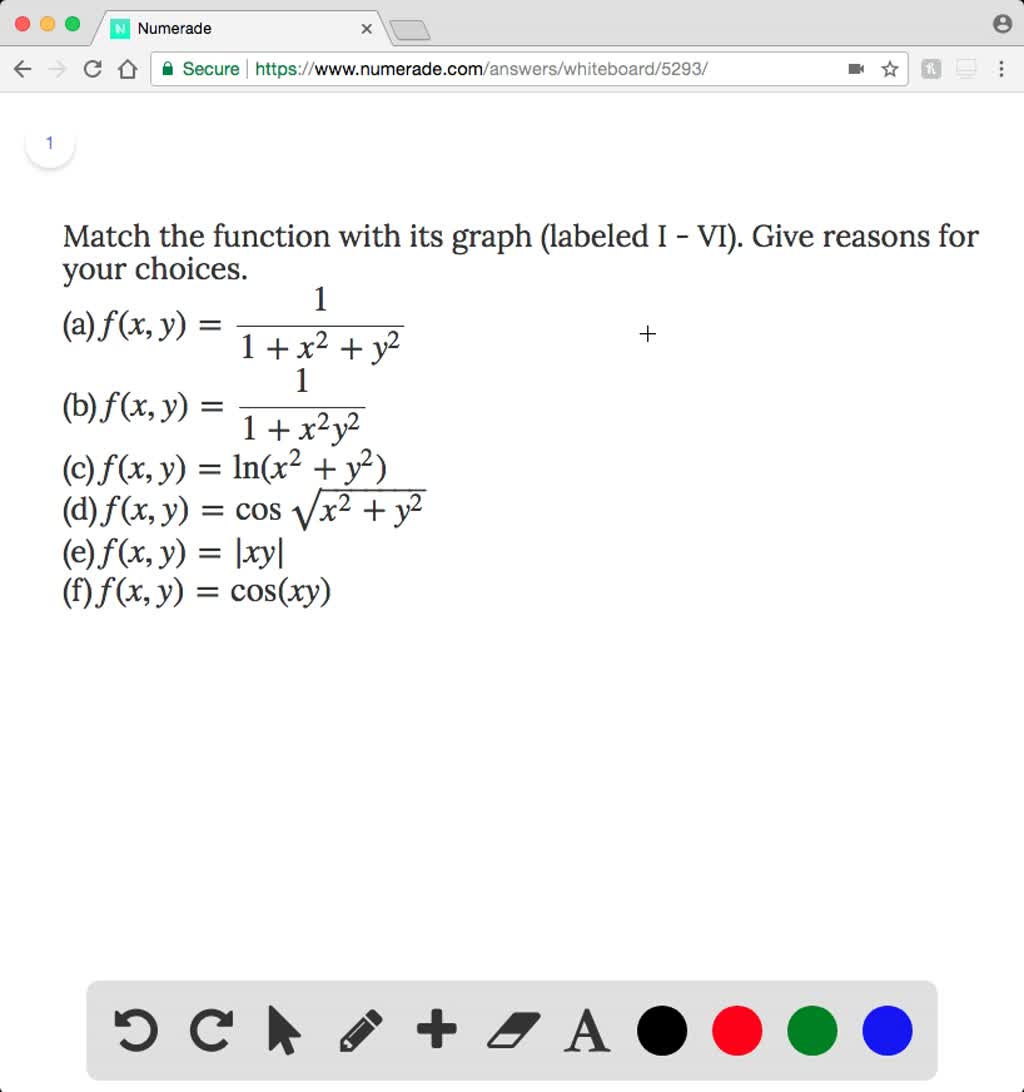


Solved Match The Function With Its Graph Labeled
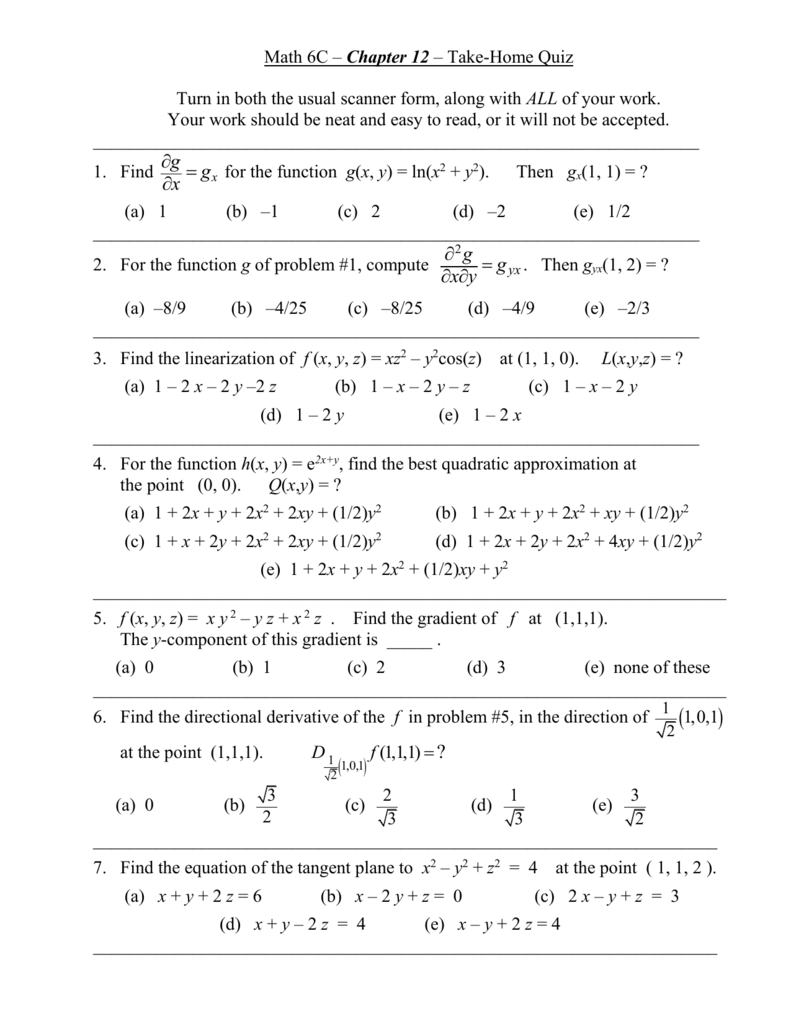


Math 6c Chapter 12 Quiz
Solution Simplify rst ln p x2 y2 = (1=2)ln(x2 y2) Then f x = (1=2)(1=(x2 y2))(2x) = x=(x2 y2) By symmetry, f y = y=(x2 y2) Di erentiating again (using the quptient rule), one determines that f xx = (x2 y2) 2x2 (x2 y2)2 = y2 x2 (x2 y2)2 By symmetry, f yy = (x2 y2)=(x2 y2)2 And so, f xx f yy = 0First of all, let us check the domain of mathy = \ln (3x^2 4x5)/math For this purpose, suppose mathf(x) = 3x^2 4x5/math Here coefficient of mathx^2=3A The given function is f(x,y)=ey in the interval x belongs to 0,8 and y belongs to 0,ln2 question_answer Q The region is formed by the graphs of y 4x and y 12x



How Do You Implicitly Differentiate Xy 2 Xcos Xy Ln X Y Ytan Xy Homeworklib
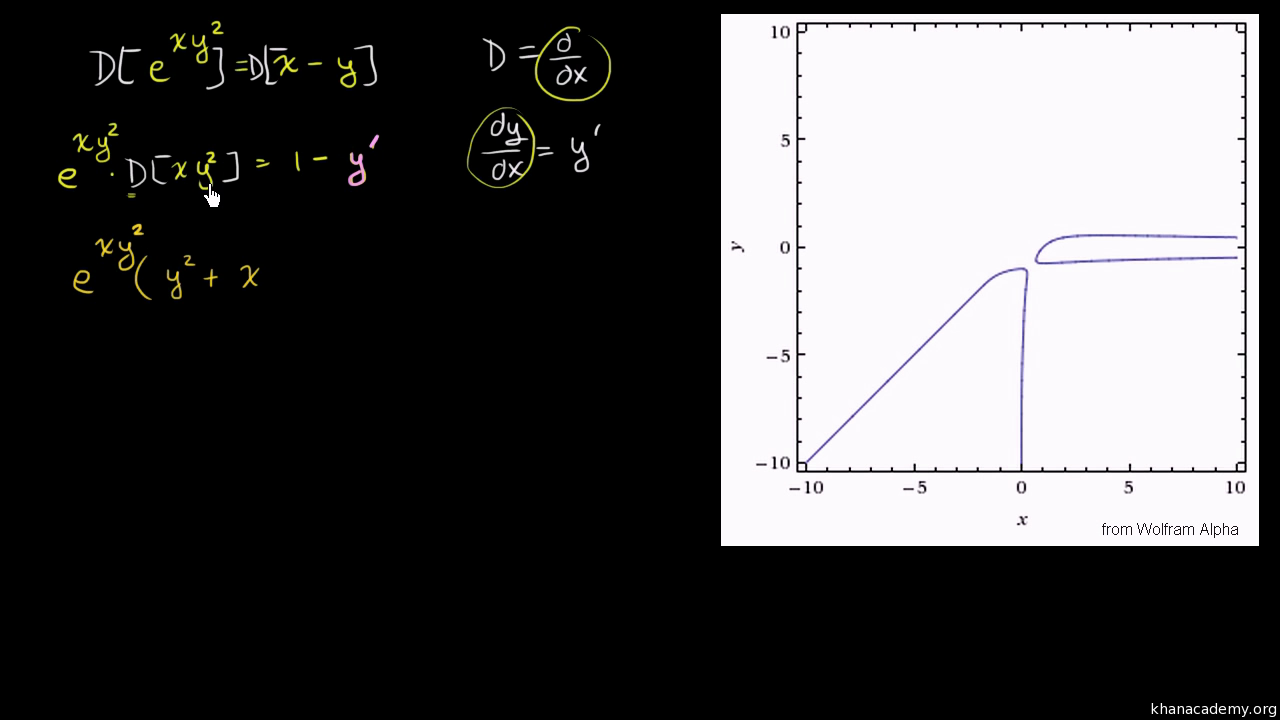


Implicit Differentiation Advanced Example Video Khan Academy
```(dz)/(dx) = 1/sqrt(x^2 y^2) (d/(dx) sqrt(x^2 y^2))` since `d/(dx) ln(f(x)) = (f'(x))/f(x)` `d/(dx) sqrt(x^2 y^2) = (1/2)(x^2y^2)^(1/2)(2x) = x/sqrt(x^2y^2)`121/x (1) ln 121/x dy dx =y − 1 x 21/xln2 121/x ln 121/x dy dx = 12 1/x x 1SeanA(x 1,y 1),B(x 2,y 2),M(3,−1)y−→v =F x y z ln x 2 y 2 z en p 111 f x y z ln x 2 y 2 z en p 111 fx zy e xy f y zx e from CALCULO II at National Open and Distance University



Show That Z Ln X 2 Y 2 2 Tan 1 Y X Satisfies The Laplaces S Equation Mathematics Stack Exchange


Ejercicios 4 Cvv Holi Matg1002 Studocu



0 件のコメント:
コメントを投稿